41 integration worksheet with answers
INTEGRAL CALCULUS - EXERCISES 45 6.2 Integration by Substitution In problems 1 through 8, find the indicated integral. 1. R (2x+6)5dx Solution. Substituting u =2x+6and 1 2
C2 INTEGRATION Answers - Worksheet A page 3 Solomon Press 9 ax + 4x2 = 0 y 10 ax2 + 2 x − 15 = 0 x(x + 4) = 0 (x + 5)(x − 3) = 0 x = −4, 0 = −5, 3 x −4 O x (−5, 0) and (3, 0) ∴ 2b = 2 ∫ 0 (x + 4x) dx = [1 b 3 x3 + x2 − 15x]3 0 = [13 x3 + 2x2]2 0 = (9 + 9 − 45) − 0 = −27 = (83 + 8) − 0 = 2 3 10 27 c
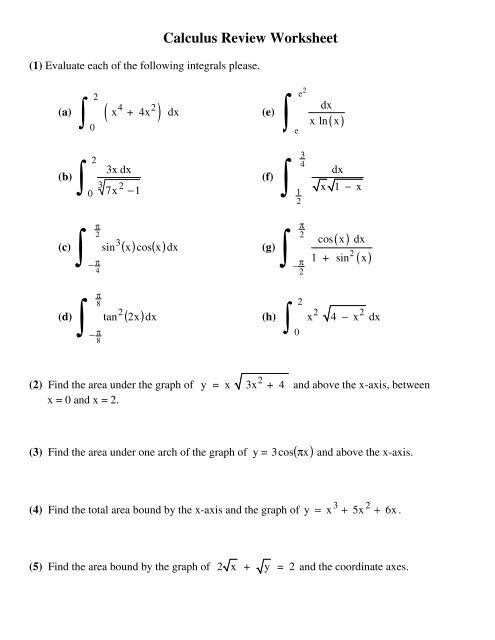
Worksheet 5.2—Mega Integration Worksheet (AB Methods) Part I: For each integral decide which of the following is needed: 1) substitution, 2) algebra or a trig identity, 3) nothing needed, or 4) can't be done by the techniques in Calculus I. Then evaluate each integral (except for the 4th type of course). A. ³ x dx3 1 4 ³x x dx23 1 x dx3 2

Integration worksheet with answers
Integration Worksheets. Integration worksheets include basic integration of simple functions, integration using power rule, substitution method, definite integrals and more. Basic Worksheets: Good practice sheets for calculus beginners. Learn the rule of integrating functions and apply it here. Basic Integration 1. Basic Integration 2
Using repeated Applications of Integration by Parts: Sometimes integration by parts must be repeated to obtain an answer. Example: ∫x2 sin x dx u =x2 (Algebraic Function) dv =sin x dx (Trig Function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx Second application ...
Section 1-1 : Integration by Parts. Evaluate each of the following integrals. ∫ 4xcos(2−3x)dx ∫ 4 x cos. . ( 2 − 3 x) d x Solution. ∫ 0 6 (2+5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1 3 x d x Solution. ∫ (3t +t2)sin(2t)dt ∫ ( 3 t + t 2) sin.
Integration worksheet with answers.
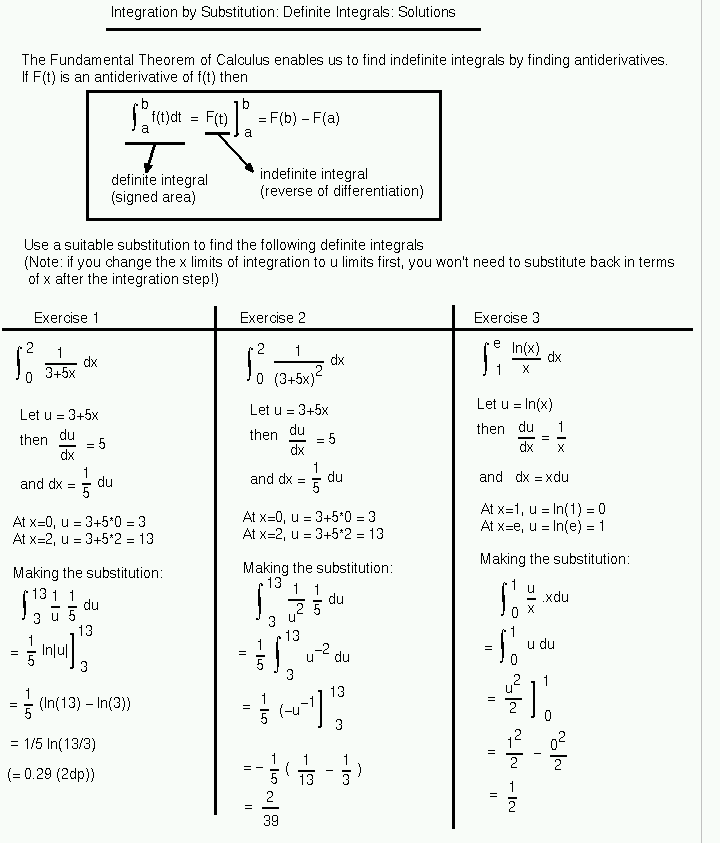
Joe Foster u-Substitution Recall the substitution rule from MATH 141 (see page 241 in the textbook). Theorem If u = g(x) is a differentiable function whose range is an interval I and f is continuous on I, then ˆ f(g(x))g′(x)dx = ˆ f(u)du. This method of integration is helpful in reversing the chain rule (Can you see why?)
CHAPTER 4/INTEGRATION MULTIPLE CHOICE. Choose the one alternative that best completes the statement or answers the question. Use a finite approximation to estimate the area under the graph of the given function on the stated interval as instructed. 1) f(x) = x2 between x = 0 and x = 3 using a left sum with two rectangles of equal width.
201-NYA-05 - Calculus 1 WORKSHEET: INTEGRALS Evaluate the following inde nite integrals: 1. Z (4x+3)dx 2. Z (4x2 8x+1)dx 3. Z (9t2 4t+3)dt 4. Z (2t3 t2 +3t 7)dt 5. Z 1 z3 3 z2 dz 6. Z 4 z7 7 z4 +z dz 7.
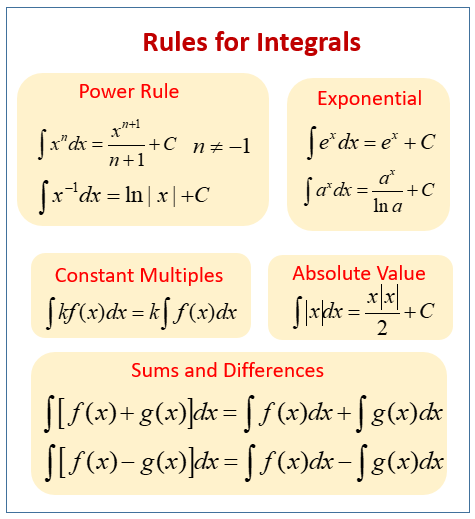
Some Basic Integration Rules: ³ 0dx C ³ kdx kx C kf x dx k f x dx³³ ªº¬¼f x g x dx f x dx g x dx r r ³ ³ ³n z 1,1 1 xn x dx C n n ³ We can also consider all the trig derivatives and go backwards to find their integrals. Examples: For each function, rewrite then integrate and finally simplify. 1. ³ 3 xdx 2. 2 1 4 dx ³ x 3. 1 dx xx ...
5.4 Exponential Functions: Differentiation and Integration Definition of the Natural Exponential Function - The inverse function of the natural logarithmic function f x xln is called the natural exponential function and is denoted by f x e 1 x. That is, yex if and only if xy ln. Properties of the Natural Exponential Function: 1. The domain of
Worksheet 5.4—Integration by Parts Show all work. No calculator unless stated. Multiple Choice 1. If ³³xxdxhx xxdxcos 2 sin 2 , then hx (A) 2sin 2 cosxx xC (B) xxC2 sin (C) 2cos sinxxx xC 2 (D) 4cos 2 sinxx xC (E) 2cos4sin xx xC2 2. ³xxdxsin 5 = (A) xx xCcos 5 sin 5 (B) 1 cos 5 sin 5 525 x
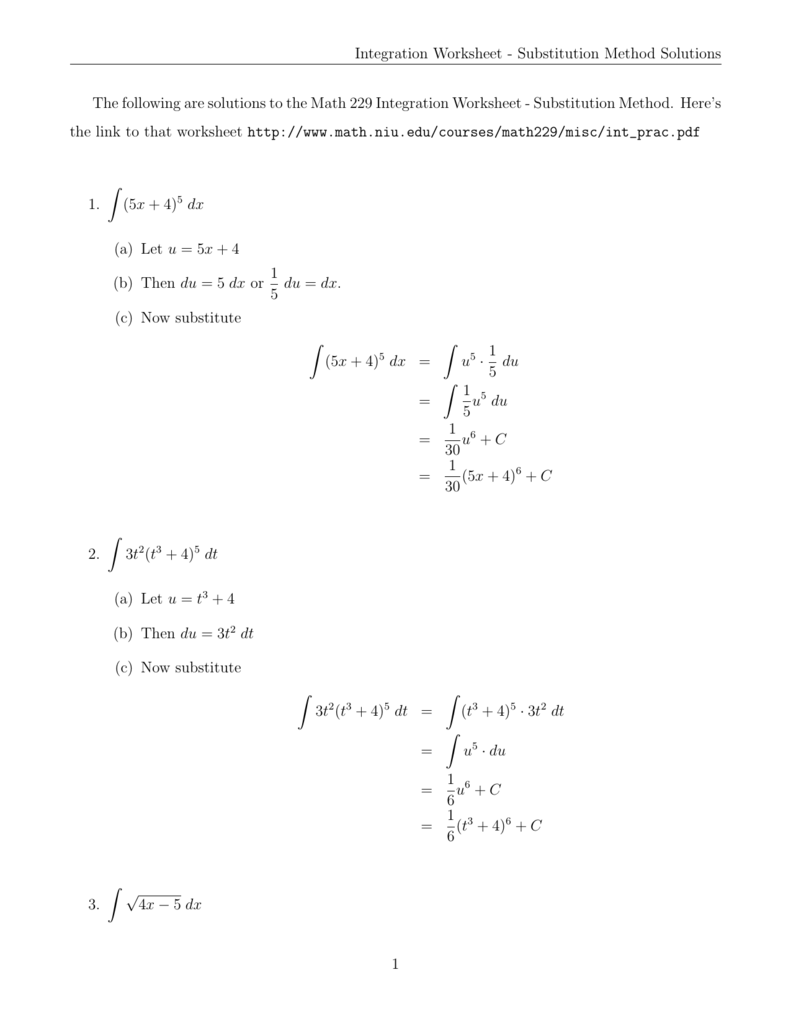
Integration Worksheet - Substitution Method Solutions (a)Let u= 4x 5 (b)Then du= 4 dxor 1 4 du= dx (c)Now substitute Z p 4x 5 dx = Z u 1 4 du = Z 1 4 u1=2 du 1 4 u3=2 2 3 +C = 1
Basic Integration Problems I. Find the following integrals. 1. (5 8 5)x x dx2 2. ( 6 9 4 3)x x x dx32 3 3. ( 2 3)x x dx 2 23 8 5 6 4. dx x xx 1 5. ( ) 3 x dx
Practice Integration Math 120 Calculus I D Joyce, Fall 2013 This rst set of inde nite integrals, that is, an-tiderivatives, only depends on a few principles of
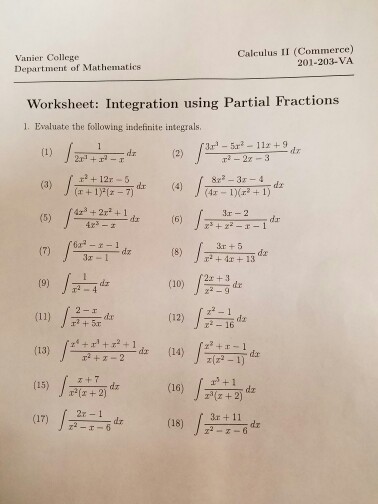
Worksheet: Integration using Partial Fractions 1. Evaluate the following inde nite integrals. (1) Z 1 2x3 + x2 x dx (2) Z 3x3 5x2 11x+ 9 x2 2x 3 dx (3) Z x2 + 12x 5 (x+ 1)2(x 7) dx (4) Z 8x2 3x 4 (4x 1)(x2 + 1) dx
Integration Methods. These revision exercises will help you practise the procedures involved in integrating functions and solving problems involving applications of integration. Worksheets 1 to 7 are topics that are taught in MATH108. Worksheets 8 to 21 cover material that is taught in MATH109.
Integration questions with answers are available here for students of Class 11 and Class 12. Integration is an important topic for 11th and 12th standard students as these concepts are further covered in higher studies. The problems provided here are as per the CBSE board and NCERT curriculum.
©T l280 L173 U ZKlu dtla M GSfo if at5w 1a4r ieE NLpL1Cs. x 9 sAXl8ln 1r FiFgDhXtLs 7 7r re As de crEv 6eVdm.2 P sMjaDd8eH pw 7i Ht4h 2 6Ian WfFiYn jiqtZe R xCKaCl2c fu Rl7u 5sm.n Worksheet by Kuta Software LLC Kuta Software - Infinite Calculus Name_____ Integration by Parts Date_____ Period____
Mixed Integration Worksheet Part I: For each integral decide which of the following is needed: 1) substitution, 2) algebra or a trig identity, 3) nothing needed, or 4) can't be done by the techniques in Calculus I. Then evaluate each integral (except for the 4 ...
Read and download free pdf of CBSE Class 12 Mathematics Integration Worksheet Set G. Students and teachers of Class 12 Integrals can get free printable Worksheets for Class 12 Integrals in PDF format prepared as per the latest syllabus and examination pattern in your schools. Standard 12 students should practice questions and answers given here for Integrals in Grade 12 which will help them to ...
Here is a set of practice problems to accompany the Computing Indefinite Integrals section of the Integrals chapter of the notes for Paul Dawkins Calculus I course at Lamar University.
©F s2Q0r1 43J GKQudt Wab WSfo sfDtvwWanrae I 8L vLuCK.C R nAkl alX Pr9i8gBhrt 2s s Nr4e msSeur 4vue hdD.G L 2M Ca2dde z Cwjiytvh M KIUn0f Gi0nWipt Qei 5CcaEluc4u FlhuQsw.Q Worksheet by Kuta Software LLC Kuta Software - Infinite Calculus Name_____ Integration Power Rule Date_____ Period____
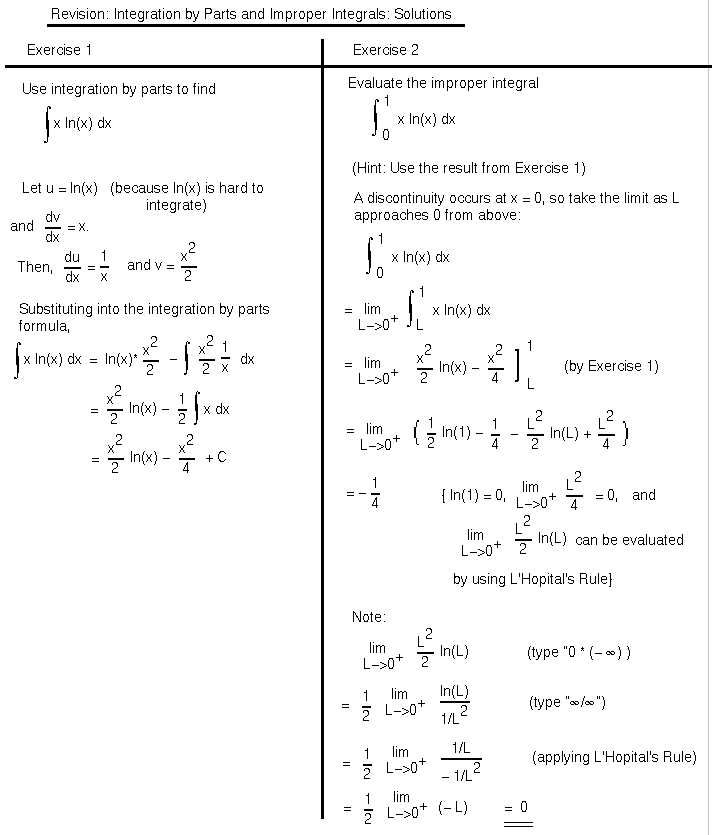
Integration by Parts To reverse the chain rule we have the method of u-substitution. To reverse the product rule we also have a method, called Integration by Parts. The formula is given by: Theorem (Integration by Parts Formula) ˆ f(x)g(x)dx = F(x)g(x) − ˆ F(x)g′(x)dx where F(x) is an anti-derivative of f(x).
13 Riemann Sums p.62-63 (Worksheet) 14 Riemann Sums p.64-65 (Worksheet) 15 Fundamental Theorem of Calculus p.66 16 Definite Integrals p.67 17 Definite Integrals p.68-71 (Worksheet) 18 Definite Integrals p.72-74 (Worksheet) 19 Review 20 TEST UNIT 7 7.1 Indefinite Integrals Calculus . Learning Objectives
5 Integration by Substitution p.52-53 6 Review for Quiz Worksheet (Passed out in Class) 7 QUIZ 1 8 Integrating Exponential Functions p.54 9 Integrating Exponential Functions p.55 -57 ( Worksheet ) 10 Integrating Rational Functions p.58 11 Integrating Rational Functions p.59 -61 ( Worksheet ) 12 QUIZ 2
Worksheet: U-Substitution Here is the truth about integration: Unlike di erentiation, all integrals are di erent and you can't just follow a formula to nd the answers. So the only way to learn how to integrate is to practice, practice, practice. Computing integrals successfully really requires you to THINK. Integrals are tricky. Examples: (1 ...
Math 129 - Calculus II. Worksheets. The following is a list of worksheets and other materials related to Math 129 at the UA. Your instructor might use some of these in class. You may also use any of these materials for practice. The chapter headings refer to Calculus, Sixth Edition by Hughes-Hallett et al. Published by Wiley.
168 Chapter 8 Techniques of Integration to substitute x2 back in for u, thus getting the incorrect answer − 1 2 cos(4) + 1 2 cos(2). A somewhat clumsy, but acceptable, alternative is something like this: Z4 2 xsin(x2)dx = Z x=4 x=2 1 2 sinudu = − 1 2 cos(u)
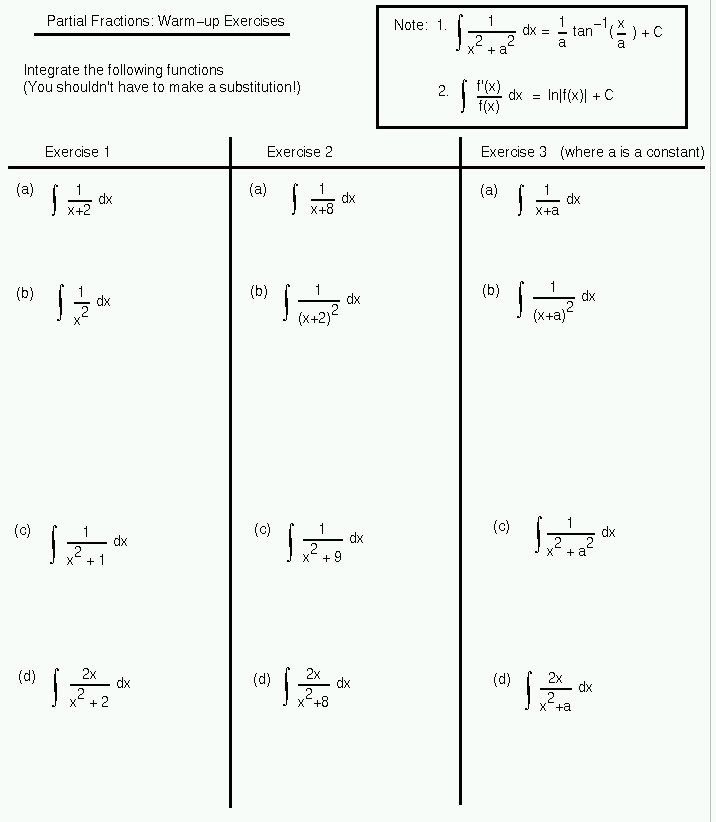




























0 Response to "41 integration worksheet with answers"
Post a Comment